Chapter 3 Part II Class Problem 1
James R. Martin, Ph.D., CMA
Professor Emeritus, University of South Florida
Chapter 3 Part II | MAAW's Textbook Table of Contents
The Crunch Company fills cereal boxes with a machine that is set to place the same amount of cereal in each box 1 . Ten samples were taken during a particular production shift that involved measuring the weight of the cereal in five boxes per sample. The results are presented below.
| Weight of Cereal in Ounces Five observations (boxes) per sample | |||||
| Sample | 1 | 2 | 3 | 4 | 5 |
| 1 | 8.41 | 7.70 | 7.90 | 7.55 | 7.92 |
| 2 | 7.68 | 8.21 | 7.58 | 7.67 | 8.09 |
| 3 | 8.69 | 7.64 | 8.16 | 8.05 | 8.15 |
| 4 | 7.48 | 8.17 | 8.50 | 7.67 | 7.66 |
| 5 | 8.38 | 7.97 | 8.05 | 7.98 | 8.31 |
| 6 | 7.48 | 8.23 | 8.23 | 7.74 | 7.75 |
| 7 | 8.29 | 8.03 | 8.21 | 7.57 | 7.93 |
| 8 | 8.00 | 7.81 | 8.33 | 7.95 | 7.76 |
| 9 | 7.90 | 7.94 | 8.07 | 8.02 | 7.31 |
| 10 | 7.52 | 7.81 | 7.93 | 8.14 | 8.07 |
Required:
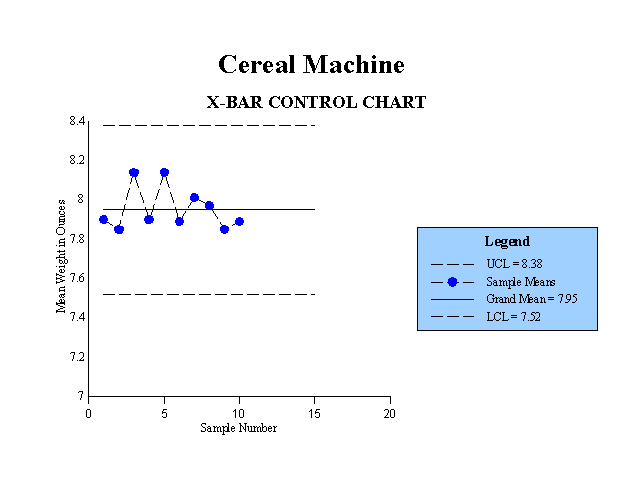
1. Determine the upper and lower limits for an X-bar chart.
2. Construct an X-bar chart.
3. Determine the upper and lower limits for a range chart.
4. Construct a range chart.
5. Does the process appear to be in control?
Explain.
6. Does the grand mean represent the same amount of cereal that the machine is designed to place in each box? Explain.
The Blank Graphs are useful for constructing the charts by hand.
| Solution |
1. Sample means and ranges are calculated in the table below.
| Weight of Cereal in Ounces Five observations per sample | ||||||||
| Sample | 1 | 2 | 3 | 4 | 5 | Total | Mean | Range |
| 1 | 8.41 | 7.70 | 7.90 | 7.55 | 7.92 | 39.48 | 7.90 | .86 |
| 2 | 7.68 | 8.21 | 7.58 | 7.67 | 8.09 | 39.23 | 7.85 | .63 |
| 3 | 8.69 | 7.64 | 8.16 | 8.05 | 8.15 | 40.69 | 8.14 | 1.05 |
| 4 | 7.48 | 8.17 | 8.50 | 7.67 | 7.66 | 39.48 | 7.90 | 1.02 |
| 5 | 8.38 | 7.97 | 8.05 | 7.98 | 8.31 | 40.69 | 8.14 | .41 |
| 6 | 7.48 | 8.23 | 8.23 | 7.74 | 7.75 | 39.43 | 7.89 | .75 |
| 7 | 8.29 | 8.03 | 8.21 | 7.57 | 7.93 | 40.03 | 8.01 | .72 |
| 8 | 8.00 | 7.81 | 8.33 | 7.95 | 7.76 | 39.85 | 7.97 | .57 |
| 9 | 7.90 | 7.94 | 8.07 | 8.02 | 7.31 | 39.24 | 7.85 | .76 |
| 10 | 7.52 | 7.81 | 7.93 | 8.14 | 8.07 | 39.47 | 7.89 | .62 |
| 79.54 | 7.39 | |||||||
The grand mean and mean of the ranges are calculated as follows:
=
X = 79.54 ÷ 10 = 7.95
_
R = 7.39 ÷ 10 = .74
Control limits for the X-bar chart are calculated as follows based on the sample size of 5 observations (See Exhibit 3-9):
=
_
X + A2R = 7.95 + (.577)(.74) = 8.38 = upper control limit.
=
_
X - A2R = 7.95 - (.577)(.74) = 7.52 = lower control limit.
2. An X-bar chart for the Cereal data is shown below.

3. Control limits for the R-chart are determined in the following manner based on the sample size of 5 observations (See Exhibit 3-9).
_
D4R = (2.115)(.74) = 1.57 = upper
control limit.
_
D3R = (0)(.74) = 0 = lower
control limit.
4. The range chart is illustrated below.
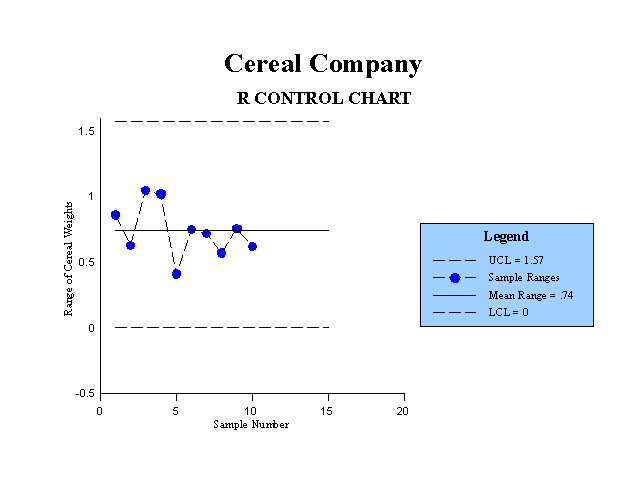
5. The process appears to be in control based on the data observed since all of the sample means and sample ranges are within the control limits.
6. The amount of cereal the machine is designed to place in each box cannot be determined from the data given. That amount could be considerably more or less than 7.95 ounces. All we can say is that the process appears to be stable and the amount of cereal placed in each box is predictable within the range given by the upper and lower limits on the R-chart.
__________________________
1 This problem was adapted from an illustration on pages 523-531 in Boot, J. C. G. and E. B. Cox. 1970. Statistical Analysis for Managerial Decisions. McGraw-Hill.